Economics is for rebels
I can guess what some of you are thinking because I used to think the same: economics is a dismal subject for bureaucrats, academics, and politicos. "The economy" is a massive, impersonal, and capricious entity that can ruin my livelihood but over which I have no control.
But what if economics were a backstop to the overreach of tyrants? What if economics were a magic lens to grasp why people behave as they do? As one of 7.9 billion humans on a planet with finite resources, aren't you a bit curious as to how we might efficiently allocate scarce resources with alternative uses across billions of people? If you feel an ounce of irreverence towards authority, or an inkling of curiosity for the causes of prosperity and cooperation, you are interested in the Austrian School of economics.
It is impossible to understand the history of economic thought if one does not pay attention to the fact that economics as such is a challenge to the conceit of those in power. An economist can never be a favorite of autocrats and demagogues. With them he is always the mischief-maker, and the more they are inwardly convinced that his objections are well founded, the more they hate him. —Ludwig von Mises [LM49]
The present article drafts insights from Ludwig von Mises and Friedrich Hayek, two of the twentieth century's most profound thinkers, to present economics in her iconoclastic glory. We will take an unexpected turn into the theory of computation that brought us the Internet, mobile phones, and the defining enterprises of our time. We will further apply the theory of computation to illuminate a nearly ubiquitous phenomenon that shapes our lives, but which few are even aware of, central planning, the persistent mischief of governments.
For the dyed in the wool students of Austrian economics among you, I will offer a deeper understanding of economic calculation, the soul of Austrian economics, through Mises's own writings. Computation, complexity, and mathematics—far from being contrivances of the present article—were essential and intentional parts of economic calculation as Mises understood it. The connection between calculation and computation persists in modern German where the verb rechnen, to calculate, is the root of the German for economic calculation (Wirtschaftsrechnung) and the noun Rechner means calculator or computer.
Computation and economics
At first glance, the suggestion that hard sciences like computer science can illuminate soft sciences like economics seems fallacious. Indeed the Austrian economists made it a point to expose physics envy, the false pretense that the human sciences can emulate the empirical methods of the hard sciences. But the theory of computation is as much about what cannot be calculated as it is about what can be calculated. And it is here, at the limits of what is computable, that we will uncover an unexpected kinship between computation and Austrian economics.
I will argue that Austrian economists view catallaxy—the market process that produces prices, exchange ratios, and the extended order of civilization—as an irreducibly complex problem that cannot be solved by central planning, but rather can only be solved by a stronger process of distributed computation that we call the market economy.
That is to say, catallactics is a process that can only execute on a distributed engine of free human agents acting in their own self-interest. Centralized bureaucratic engines—be they committees of experts, politicians, scientific models, or well-defined procedures of any kind—are structurally incapable of economic calculation and therefore incapable of producing the order, abundance, and prosperity that characterize the developed world.
The duality of science and economics
I am not arguing, nor do I believe, that the theorems of computer science apply to humans. I will take the Austrian position that the methodology of the physical sciences isn't even valid for economics. Mises emphatically separates the hard sciences from economics, denouncing "quantitative economics" as a barking cat:
In the realm of physical and chemical events there exist (or, at least, it is generally assumed that there exist) constant relations between magnitudes, and man is capable of discovering these constants with a reasonable degree of precision by means of laboratory experiments. No such constant relations exist in the field of human action outside of physical and chemical technology and therapeutics... Those economists who want to substitute "quantitative economics" for what they call "qualitative economics" are utterly mistaken. There are, in the field of economics, no constant relations, and consequently no measurement is possible. [LM49]
As we proceed it will be instrumental to understand how the Austrian methodology of economics is not only distinct from the methodology of science, but how the two methodologies are logical duals. Table 1 summarizes this duality and reveals that the scientific and Austrian perspectives are opposite poles of an epistemological spectrum.
| Physical sciences | Austrian economics |
|---|---|
| Physical evidence | Logical arguments |
| A posteriori knowledge | A priori knowledge |
| Empirical, positivist | Praxeological |
| Quantitative | Qualitative |
| Constants | Variables only |
| Controlled experiments | Imaginary constructions |
| Inductive | Deductive |
| Hypothesize behavior of parts from observation of whole | Deduce behavior of whole from knowledge of parts |
Table 1 — The duality of the physical sciences and Austrian economics
Complexity is the unity above the duality
It is possible to at once respect the duality of science and Austrian economics, and simultaneously zoom out to a level of generality where we can see the common properties of both systems. We will find that the structure and patterns of computation bring us appreciation and intuition for Austrian economics, and vice versa. We may therefore take examples of the theory of computation not as facts about human action, but as imaginary constructs of pure logic that we can use to deduce, as praxeologists do, what is eternally true about the world. Just as scientists test their ideas in controlled experiments in the laboratory, the praxeologist tests imaginary constructs in the laboratory of the mind.
The praxeological core of Austrian economics and Turing's theory of computation are both formal systems.
A formal system is an abstract structure used for inferring theorems from axioms according to a set of rules. [WFS22]
Moreover the formal systems of praxeology and computation describe organic realities known as complex systems. Economies and computer programs are both complex systems in that they consist of numerous parts that interact to produce structured but rarely predictable wholes.
A complex system is a system composed of many components which may interact with each other. Examples of complex systems are Earth's global climate, organisms, the human brain, infrastructure such as power grid, transportation or communication systems, complex software and electronic systems, social and economic organizations (like cities), an ecosystem, a living cell, and ultimately the entire universe. [WCS22]
The study of complex systems encompasses an incredibly broad family of knowledge including game theory, nonlinear dynamics, evolution, networks, information theory, and much more (Figure 1).
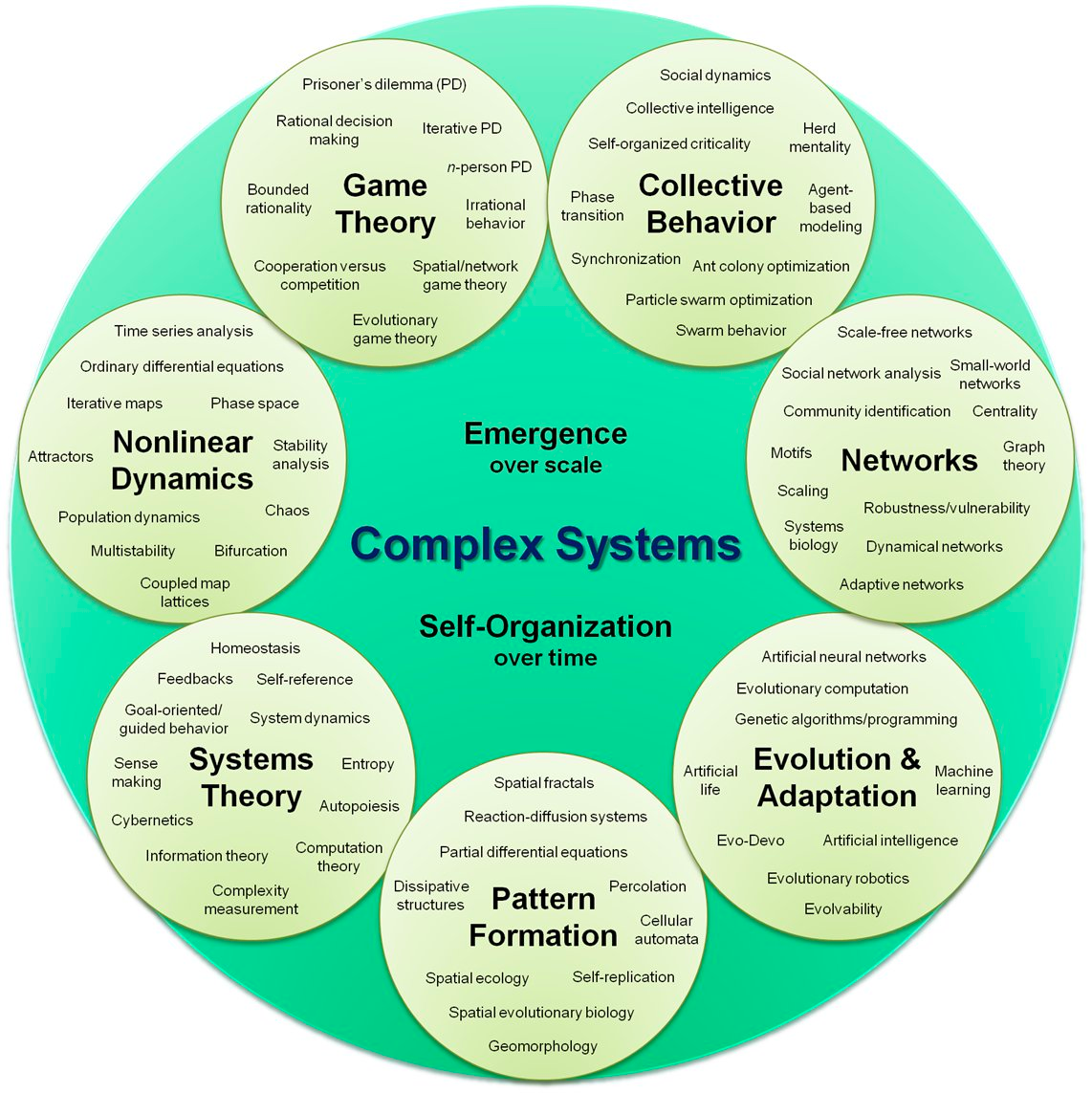
We will pay special attention to three key features of complex systems: emergence, self-organization, and unpredictability. Unpredictability implies that, even if the initial conditions of a complex system are known, the outcomes and future states of that system are unknown. As a result of their unpredictability, complex systems are difficult or impossible to model, a fact that we will revisit repeatedly as we dive into Mises's economic calculation problem. In brief, the economic calculation problem is Mises's argument as to why socialist economic systems must fail: they are computationally incapable of providing acting humans with objective facts about the relative merits of alternative courses of action. If we read Mises carefully, we hear him describe the necessary bureaucracy of socialist economies as a mindless automaton, not unlike a giant computer:
All transactions… will be subject to the control of a supreme authority. Recourse will be had to the senseless output of an absurd apparatus. The wheels will turn, but will run to no effect. [GS13]
Figure 2 shows a complex system that has been the subject of of hundreds of scientific articles and even more popular science articles—indulge me for a moment—the Lorenz attractor. In spite of the fact that you can implement a Lorentz attractor in a dozen lines of code, it hides tremendous nonlinearity: minuscule differences in the starting conditions of a Lorenz attractor lead to radically different outcomes. Nonlinearity has popularly been called the butterfly effect:
In any physical system, in the absence of perfect knowledge of the initial conditions (even the minuscule disturbance of the air due to a butterfly flapping its wings), our ability to predict its future course will always fail. This underscores that physical systems can be completely deterministic and yet still be inherently unpredictable... [WLS22]

Let's examine the paradox in the second sentence above, "physical systems can be completely deterministic and yet still be inherently unpredictable." This carries us straightaway to the methodology of Austrian economics, praxeology. Although the axioms of praxeology and human behavior are simple and few, the precise social outcome that results from the interaction of numerous human agents is unknowable to anyone, including central planners armed to the gills with models, data, and every conceivable science. Isn't it ironic that mathematical models in a handful of variables are complex enough to defy prediction, and yet armies of quantitative economists try to pretend that simplistic equations could hope to capture complex human phenomena like employment, interest, and money?
Before we dive further into technical details, let's develop our intuition for complex systems with a page from the book of nature, the murmuration of starlings.
Emergence and the extended order
Take a moment to appreciate the beauty of flocking starlings, a phenomenon known as murmuration (Figure 3). Observe how a complex and seemingly orchestrated dance emerges between hundreds, thousands, or even millions of birds [AT19]. And yet there is no conductor, no mastermind, and no central plan behind this organic order. Each literally bird-brained agent holds only fleeting local information in its head. We call this phenomenon a higher order, because it arises from a simple lower order, the survival imperative to stay in the air.
A constructive proof that the higher order of murmuration arises from lower order rules comes from a simulation technique called Boids, devised by my colleague Craig Reynolds in 1986 [WB22]. Craig showed that just three simple rules produce phenomena that match real-world congregations of birds, fish, zebra, humans—pretty much any social animal. Each bird or agent in a Boids simulation does only three things:
- separation — don't crash into your neighbors
- alignment — fly in roughly the same direction as your neighbors
- cohesion — stay close to your neighbors
These three simple and purely local rules, distributed across the agents of the simulation, interact to generate the complex holistic phenomenon of murmuration. (Curious readers can experiment with the three rules of Boids with this online simulation.)
Murmuration emerges, without any central planning, from the interaction of autonomous agents with purely local knowledge. Starlings don't plan murmuration, it happens. Similarly, Adam Smith's often-misunderstood invisible hand is nothing mystical. The invisible hand denotes the process by which selfish human actions lead to globally rational outcomes (by a mechanism which is unknown but nevertheless concrete and measurable). Smith offers the example of a consumer who selfishly prefers local goods for the purpose of strengthening his own economic security:
He generally, indeed, neither intends to promote the public interest, nor knows how much he is promoting it. By preferring the support of domestic to that of foreign industry, he intends only his own security; and by directing that industry in such a manner as its produce may be of the greatest value, he intends only his own gain, and he is in this, as in many other cases, led by an invisible hand to promote an end which was no part of his intention... By pursuing his own interest he frequently promotes that of the society more effectually than when he really intends to promote it. —Adam Smith [WAS22]
We are now ready to glimpse the wonder of what Hayek calls the extended order, the distributed process that organizes civilization and generates wealth as we know it. Here Hayek argues that the extended order can never be the byproduct of central planning:
The curious task of economics is to demonstrate to men how little they really know about what they imagine they can design. To the naive mind that can conceive of order only as the product of deliberate arrangement, it may seem absurd that in complex conditions order, and adaptation to the unknown, can be achieved more effectively by decentralizing decisions and that a division of authority will actually extend the possibility of overall order. Yet that decentralization actually leads to more information being taken into account. —Friedrich Hayek [FH11]
The Fatal Conceit is not only the title of Hayek's book and the source of the above quotation, but a demonstrable flaw in the thinking of all socialists. The fatal conceit of socialists and central planners throughout history is the belief that order always and only results from conscious and deliberate design. This must be a fallacy since the extended order requires not only rationality but distributed information and individual morality (religion, tradition, intuition) in the broadest sense of the word. With acumen, Hayek challenges the conceit of those in power not by attacking their persons or even their arguments, but by demolishing the presumption on which they stand, the pretense of greater knowledge. We'll revisit the fatal conceit when we dive into the algorithmic nature of central planning.
The absence of central planning is not chaos
Armed with Hayek's observation that higher orders emerge, we pause to address a common objection to laissez-faire economics: without central coordination there is pandemonium. The false dichotomy beneath this objection is the belief that the absence of central planning is no planning whatsoever. But in fact, as central planning disappears, distributed (or decentralized) planning takes its place. Distributed planning—far from being blind or chaotic—is planning par excellence, for distributed planning incorporates more information and more skin in the game than its centralized alternative.
What is called a planned economy is no economy at all. It is just a system of groping about in the dark. There is no question of a rational choice of means for the best possible attainment of the ultimate ends sought. What is called conscious planning is precisely the elimination of conscious purposive action. —Ludwig von Mises [LM49]
Mises understood that "production anarchy" was not actually the state of affairs under capitalism, but a superficial smear leveled at the market by autocrats and demagogues who seek to replace distributed planning with their own designs:
When the socialists declare that "order" and "organization" are to be substituted for the "anarchy" of production, conscious action for the alleged planlessness of capitalism, true cooperation for competition, production for use for production for profit, what they have in mind is always the substitution of the exclusive and monopolistic power of only one agency for the infinite multitude of the plans of the individual consumers and those attending to the wishes of the consumers, the entrepreneurs and capitalists. [LM49]
From starlings to praxeology
Before we depart from our sky full of starlings, we must learn what starlings have to teach us about the methodology of Austrian economics. Consider a single starling, alone on a power line before she takes flight to join her companions in murmuration. While it is impossible to predict where our starling will be after a few seconds of murmuration, and while it is impossible to predict the higher order shapes that our starling and her peers will produce as they flock, we can nevertheless say with certainty that our starling will be somewhere within a higher order structure that is absolutely unique to history and yet commonly and everywhere recognizable as murmuration. Though the form of this higher order structure is wholly unknown to us, its future existence is certain. We stake the certainty of our belief that the starling will be somewhere in an unknown but recognizable murmuration on the knowledge that each and every starling follows simple and universal rules.
Just as starlings unintentionally generate spectacular order from simple rules of behavior, Austrian economists hold that the complex order of society derives from simple and eternal rules of human behavior, captured in the axioms of praxeology. The epistemological direction of praxeology is therefore dual to that of science, as shown in Table 1. Praxeology deduces the behavior of the whole from known parts, the axioms of human action. The axioms of human action, Mises argues, are eternal and unchangeable functions of the structure of man's mind and the structure of man's environment. The first and most important of these rules is a priori and can be arrived at by self-examination. It is known as the axiom of action.
Praxeology rests on the fundamental axiom that individual human beings act, that is, on the primordial fact that individuals engage in conscious actions toward chosen goals. This concept of action contrasts to purely reflexive, or knee-jerk, behavior, which is not directed toward goals. The praxeological method spins out by verbal deduction the logical implications of that primordial fact. In short, praxeological economics is the structure of logical implications of the fact that individuals act. This structure is built on the fundamental axiom of action, and has a few subsidiary axioms, such as that individuals vary and that human beings regard leisure as a valuable good. —Murray Rothbard [MR19]
Above, Rothbard uncovers the formal core of praxeology. Starting from a few axioms, the Austrian economist deduces the nature of the whole (society) based on certain knowledge of its parts (humans). Setting aside for a moment whether or not praxeology is at all valid, would it not be folly to attempt to understand the world from the whole down to its parts? How could one possibly start with the whole of society and attempt, from this bewildering height of complexity and the multitudinous facts of history, to arrive at an understanding of human nature? Yet this is what John Maynard Keynes, whom we shall meet later under the ludic fallacy, and his descendants do: propose oversimplified and scientistic equations to model irreducibly complex wholes. How can such economists even pretend to follow the scientific method of controlled experiments in a world of constant change, billions of diverse actors, uncountable hidden variables, and imperfect knowledge? Which of these uncountable variables do quantitative economists pretend to have the power to control to even begin to prove their theories of economics?
The axioms of praxeology
Somewhat strangely—have I missed something?—I have yet to find the axioms of praxeology neatly captured in a single, authoritative list. For the reader's benefit, I include the axioms of praxeology as I understand them below. The axiom of action is primary in that the other axioms follow from it to a greater or lesser degree.
- Humans engage in purposeful action to attain goals
- Humans are highly diverse
- Humans act to reduce an inner uneasiness
- Human action is fraught with uncertainty
- Humans engage in exchange to meet their goals
- Humans value leisure
Wait, what did he just say?
When I first discovered Austrian economics I found its claims overreaching, bordering on the absurd. How could such a simple and self-contained system—one that evades falsification and denies the importance of empirical evidence—lead to certain conclusions about human action and economics? On what basis do Austrians claim that the axioms of praxeology are eternal and unchangeable? It is not my intention to answer these questions for you in a single essay. Rather, I present these topics for your consideration, in the light of computation, so that you can inquire as to the truth for yourself. I would direct the curious student to Mises's magnum opus, Human Action for a full philosophical, epistemological, and economic analysis of the dangers of socialism. Human Action is as erudite and rewarding as it is long, so buckle up.
We're not done yet. I've just paused to acknowledge that Austrian economics is deep, counterintuitive, and sometimes maddening to ponder. Grab a beverage of your choice and come back. We're going to work our way towards the heart of Austrian economics, economic calculation, where the theory of computation has much to teach us.
Algorithms and economics
Mises defines the market not as a place or thing, but as a process.
The market is not a place, a thing, or a collective entity. The market is a process, actuated by the interplay of the actions of the various individuals cooperating under the division of labor. [LM49]
It as at the level of a complex process that we find a pivotal connection between computation and economics. The market is a process that transforms human action into prices and products. Similarly, a computer executes processes that transforms inputs into outputs. Nevertheless, the market is not what computer scientists would call algorithmic. An algorithm is a step-by-step procedure for solving a problem. Traditionally, algorithms have been thought of as instructions for computers. But algorithms describe the functioning of bureaucracies with startling accuracy:
You will never understand bureaucracies until you understand that for bureaucrats procedure is everything and outcomes are nothing. —Thomas Sowell
Anyone who has programmed a computer can tell you that Sowell's description of bureaucracies is uncannily apt for computers: You will never understand computers until you understand that for computers procedure is everything and outcomes are nothing. Bureaucrats and computers alike blindly follow syntactical procedures with no understanding of human semantics or intentions.
Any central plan that is not algorithmic must be unfair, for its realization relies not on well-defined procedures but on the whims and discretion of central planners. Therefore central plans are either unfair by virtue of the bias and blindness of their algorithms (all models are wrong) or unfair by virtue of the whims of the executors of the plan. Mises, Sowell, and Rothbard all concur that socialist bureaucracies exhibit the character of machines. Nevertheless, we must split the hair that computer algorithms are executed by Turing machines, whereas bureaucratic algorithms are executed by people. We therefore use algorithm in an extended sense to capture the mechanistic nature of computers and socialism alike.
Although the market economy, catallaxy really, is a process, it is not algorithmic in that there are no steps that we can write down that apply to the economic calculation of every individual in the economy. Nevertheless, the Austrian view of economic calculation is, we shall see, computational. Austrian economics concerns itself solely with the means of economic calculation and leaves aside the ends, a posture of wertfreiheit.
The wertfreiheit of computation
Praxeology concerns itself purely with the morally neutral means that acting man applies to achieve his ends. The German language has a unique word for this morally neutral posture, wertfrei. (The closest phrase in English is something like amoral and clinical). Mises describes wertfreiheit:
In this sense we may say that economics is apolitical or nonpolitical, although it is the foundation of politics and of every kind of political action. We may furthermore say that it is perfectly neutral with regard to all judgments of value, as it refers always to means and never to the choice of ultimate ends. [LM49]
Computation, like economic calculation, makes no thought, indeed cannot think, about the moral content of its programs, but is merely a means to human ends. The link between economic calculation and computation runs deep. Mises observes:
Computation demands units. And there can be no unit of the subjective use value of commodities. Marginal utility provides no unit of value…Judgments of value do not measure: they arrange, they grade. [GS13]
Mises is ever exact and deliberate with his choice of words. Computation—harkening again to the blurry line between calculation and computation in German—is clear enough. But we must take a moment to unpack subjective use value, marginal utility, and the extraordinary statement that "Judgments of value do not measure: they arrange, they grade." Mises is explaining that value judgments are ordinal but not cardinal. Ordinal and cardinal are not only Mises's own words in Human Action, which he wrote in English, but are foundational to mathematics and computer science.
In order to understand the distinction between cardinality and ordinality, we must journey back to the father of Austrian economics, Carl Menger, who resolved the centuries-old diamond-water paradox.
Marginal utility and the diamond-water paradox
Dating as far back as Plato, the diamond-water paradox (or paradox of value) poses the question of why diamonds cost more than water when the latter is clearly more essential to human existence [WPV22]. The brilliant Adam Smith introduced the distinctions of use value and exchange value to explain the paradox. (Diamonds have low use value but high exchange value, and vice versa for water.) But Smith was too clever for his own good. Smith unwittingly created a second paradox in the process of shattering the monad of value into two halves [GS13]. If goods can be viewed along two conflicting dimensions of value, which of these two values does acting man choose in making economic choices?
Menger resolved the diamond-water paradox with the breakthrough concept of marginal utility. Marginal utility demolishes the duality of the diamond-water paradox by observing that human beings do not exchange or purchase objects because of their general value. Rather, acting man calculates the value of his purchases based on their specific value to him at the unique and idiosyncratic moment of purchase. A woman in the desert dying of thirst values a gallon of water more than any number of diamonds. A man about to marry may value one diamond more than many gallons of water.
Table 2 contradistinguishes the two dimensions of value. Marginal utility, we note, breaks the duality of the subjective and objective dimensions of value by recognizing that specific values must transact at market prices.
| Personal preferences | Market price |
|---|---|
| Ordinal numbers | Cardinal numbers |
| Specific value | General or exchange value |
| Value | Price |
| Subjective | Objective |
| Intensive | Extensive |
Table 2 — Subjective and objective dimensions of price and value
Economic calculation
Economic calculation is the heart of Mises's philosophy. He observes that
Economic calculation is the fundamental issue in the comprehension of all problems commonly called economic. [LM49]
We can briefly define economic calculation as the process by which acting man evaluates alternatives to best satisfy his goals. A hungry man may, for reasons unknown to us, prefer blueberries over beef to ease his hunger. His preferences form an ordinal hierarchy that express his relative preferences:
- Blueberries
- Beef
In his mind, blueberries are first and beef is second. But it's nonsense to say that "he prefers beef half as much as blueberries". The coincidence that one divided by two equals one-half is devoid of actionable meaning. In order to pacify his hunger in an exchange-driven society, our actor must compare the costs of acquiring his aims on an objective and cardinal scale. While ordinal numbers indicate subjective preferences, cardinal numbers convey objective quantities. Price is a cardinal value:
7$ for one pint of blueberries
5$ for one pound of beef
The signal of price provides acting man with the means to evaluate precisely how much he prefers blueberries to beef. Here our famished actor must choose if he prefers two dollars in his pocket or a pint of blueberries in his stomach.
The difference between the value of the price paid (the costs incurred) and that of the goal attained is called gain or profit or net yield. Profit in this primary sense is purely subjective, it is an increase in the acting man’s happiness, it is a psychical phenomenon that can be neither measured nor weighed. There is a more and a less in the removal of uneasiness felt; but how much one satisfaction surpasses another one can only be felt; it cannot be established and determined in an objective way. —Ludwig von Mises [LM49]
The ordinal, the cardinal, and the economic calculation problem
Now that we understand the difference between subjective values (ordinal numbers) and objective prices (cardinal numbers) we are poised to understand one of Mises's strokes of genius, the economic calculation problem. Before the unforgettable collapses of the USSR, Venezuela, and other socialist economic systems, Mises had already predicted their demise decades earlier. How did Mises accomplish this act of fortune telling? Socialist economies cannot by definition perform economic calculation because there is no algorithmic process for turning subjective ordinal numbers into objective cardinal numbers. For ordinal preferences are purely subjective and essentially dispersed knowledge in the hearts of acting humans. In the absence of a market, socialist managers have no means to convert their subjective preferences into objective and actionable plans. In a socialist economy of public ownership, no real exchange takes place, there is merely an internal transfer of goods [WECP22].
We can now rephrase the fatal conceit in computational terms. The fatal conceit of socialists is the mistaken belief that algorithmic procedures can satisfy the material needs of mankind. And the reason that socialists are forever caught dodging the evidence of history with the four most expensive words in the English language, "this time it's different", is that they are stuck in their algorithmic worldview. They think to themselves "we now have more data, technology, and science than ever before." Although that may be the case, this does not change the fundamental fact that no centralized, rational procedure is even capable of generating the extended order. Because the extended order requires not only economic calculation, of which socialist nations are incapable, but enormous numbers of received behaviors that survived through a process of social evolution—religion, trade secrets, morals—that guide human action but for which no rational justification is even possible. Moreover the acting "man on the spot", as Hayek calls him, possesses timely and urgent information for action that he cannot possibly convey to any central planner [FH45].
Imagine, for a moment, how impossible and treacherous driving a car would become if you had to phone a bureaucracy (only open Monday thru Friday from 9 to 5, except on holidays) for permission before each press of the brakes or accelerator. Or how quickly a starling would fall from the sky if it had to wait even a fraction of a second for someone's permission to act.
The most common denominator (and spiders on marijuana)
In order for economic calculation action to address and coordinate a nearly infinite variety of goods and services, it requires a common denominator. Mises elucidates:
It is not the task of economic calculation to expand man’s information about future conditions. Its task is to adjust his actions as well as possible to his present opinion concerning want-satisfaction in the future. For this purpose acting man needs a method of computation, and computation requires a common denominator to which all items entered are to be referable. The common denominator of economic calculation is money. [LM49]
We must recognize here that not only consumer goods are the elements of human calculation, but so are higher-order goods, also known as capital goods. Higher-order goods are the goods used to make consumer goods. For instance, Apple acquires the higher-order goods of plastic, glass, aluminum, and microprocessors to produce the iPhone.
Leonard Read's inimitable I, Pencil—a short essay beloved by Milton Friedman—vividly demonstrates the bewildering complexity of consumer goods as simple as a pencil. Indeed, the manufacture of a pencil is so complex that no single person on Earth knows everything about how to construct one [LRI15]:
My cedar receives six coats of lacquer. Do you know all the ingredients of lacquer? Who would think that the growers of castor beans and the refiners of castor oil are a part of it? They are. Why, even the processes by which the lacquer is made a beautiful yellow involve the skills of more persons than one can enumerate!
Read continues
My bit of metal—the ferrule—is brass. Think of all the persons who mine zinc and copper and those who have the skills to make shiny sheet brass from these products of nature. Those black rings on my ferrule are black nickel. What is black nickel and how is it applied? The complete story of why the center of my ferrule has no black nickel on it would take pages to explain. [LRI15]
Read goes on to talk about the rubber, the graphite, the factory and the myriad factors of labor, production, and technology that conspire to produce a humble pencil. I, Pencil demonstrates an unimaginably complex and intertwingled system of prices that guide human action. Indeed not just consumer prices but the prices of every higher-order good derive from economic calculation:
Menger’s discussion of higher order goods allowed him to apply the notion of marginal utility not only to consumer goods but to the factors of production as well. This insight proved essential to the Misesian argument that planners in a socialist economy will be unable to engage in rational economic calculation. —George Smith [GS13]
It must therefore be the case that errors in the denominator of economic calculation, money, have catastrophic butterfly effects on the economy as a whole.
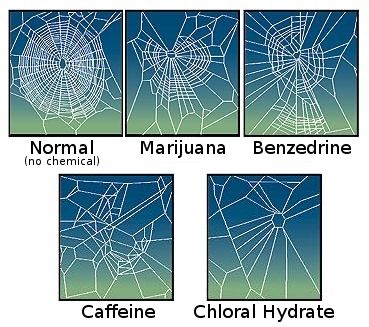
Here we may speculate that if Bitcoin emerges as a currency that is free from arbitrary inflation by bureaucrats, it would allow for a clarity of economic calculation that is unprecedented in history. Economic calculation requires two things: a market, and a medium of exchange [WEC22]. If we think of fiat money, the present denominator of economic calculation, as a ruler whose length arbitrarily changes by the minute, we grasp the distorting effects that inflation has on economic calculation. To push the analogy, it would be impossible to build skyscrapers if every architect, engineer, and supplier had slightly different definitions of a meter. Buildings beyond a few stories would slowly accumulate enough small errors as to be wholly unstable. The distortionary effects of fiat currency on economic calculation remind us of the effective of psychoactive drugs on animals (Fig. D, [WEPDA22]). Just as naval travel blossomed from the invention of steel, and air travel flourished from the invention of aluminum, what new economic structures might be possible if the doors of monetary perception were cleansed?
Read finishes
The lesson I have to teach is this: Leave all creative energies uninhibited. Merely organize society to act in harmony with this lesson. Let society’s legal apparatus remove all obstacles the best it can. Permit these creative know-hows freely to flow. Have faith that free men and women will respond to the Invisible Hand. This faith will be confirmed. I, Pencil, seemingly simple though I am, offer the miracle of my creation as testimony that this is a practical faith, as practical as the sun, the rain, a cedar tree, the good earth. [LRI15]
What about market socialism?
The problem of economic calculation was serious enough for socialist economists to take notice. Admitting that economic calculation and price discovery were essential features of civilization, they proposed a form of market socialism captured in the Lange-Lerner-Taylor model. In brief, market socialism proposes that, just as capitalists discover prices by "trial and error", socialist managers could try different prices until the correct price was discovered. This is absurd for three reasons: socialist managers have no skin in the game, socialist managers lack knowledge of what is happening on the ground, and socialist managers have neither the time nor the dynamism nor the capacity to continually adjust prices up to the moment as markets do. Mises shows prescient understanding of skin in the game in Human Action:
One cannot play speculation and investment. The speculators and investors expose their own wealth, their own destiny. This fact makes them responsible to the consumers, the ultimate bosses of the capitalist economy. If one relieves them of this responsibility, one deprives them of their very character. [LM49]
Neither is there any algorithmic curriculum by which a socialist economy could train entrepreneurs, to say nothing of socialist managers with no skin in the game. Entrepreneurs, I note, are a fundamental and driving force of the economy in the Austrian School:
An entrepreneur cannot be trained. A man becomes an entrepreneur in seizing an opportunity and filling the gap. No special education is required for such a display of keen judgment, foresight, and energy. The most successful businessmen were often uneducated when measured by the scholastic standards of the teaching profession. But they were equal to their social function of adjusting production to the most urgent demand. Because of these merits the consumers chose them for business leadership. [LM49]
Believing unflinchingly as he did in the power of central planning, Oskar Lange (of Lange-Lerner-Taylor fame) claimed that what socialist economies would need to overcome the economic calculation problem is—wait for it—a computer:
Were I to rewrite my essay ["On the Economic Theory of Socialism"] today my task would be much simpler. My answer to Hayek and Robbins would be: so what's the trouble? Let us put the simultaneous equations on an electronic computer and we shall obtain the solution in less than a second. The market process with its cumbersome tâtonnements appears old-fashioned. Indeed, it may be considered as a computing device of the pre-electronic age. [MR91]
Rothbard, in some sense the spiritual successor to Mises, replies:
Lange's naive enthusiasm for the magical planning qualities of the computer in its early days can only be considered a grisly joke to the economists and the people in the socialist countries who have seen their economies go inexorably from bad to far worse despite the use of computers. Lange apparently never became familiar with the computer adage, GIGO ("garbage in, garbage out"). Nor could he have become familiar with the recent estimate of a top Soviet economist that, even assuming that the planning board and its computers could learn the correct data, it would take even the current generation of computers 30,000 years to process the information and allocate the resources. [MR91]
What Lange failed to understand was that market prices are not merely difficult to compute, but downright impossible to compute. (For this reason Rothbard takes Hayek and his differential equations to task for softening the impossibility of Mises's economic calculation problem into something that was merely intractable [MR91].) To appreciate the distinction between intractable and impossible we must turn to the father of computer science, Alan Turing.
Turing, Chomsky, and the incomputable
Alan Turing invented one of the earliest models of computation, now known as the Turing machine. A Turing machine is a formal system that manipulates symbols on an infinite tape according to a set of rules. That word "infinite", by the way, is why you aren't reading this article on a Turing machine but on a linearly bounded automaton (LBA), which is similar to a Turing machine but has finite memory. Table 3 shows the Chomsky hierarchy of automata.
| Grammar | Languages | Automoton |
|---|---|---|
| Type-0 | Recursively enumerable | Turing machine |
| Type-1 | Context-sensitive | Linear-bounded non-deterministic Turing machine |
| Type-2 | Context-free | Non-deterministic pushdown automaton |
| Type-3 | Regular | Finite state automaton |
Table 3 — Chomsky's hierarchy of automata [WCH22]
Turing gave the world not only early theoretical models of computation, but showed that certain questions simply cannot be answered by computers. One of these questions is the halting problem. We've all had computers freeze on us, right? You can think of a frozen computer stuck in an infinite loop as a special case of halting. Turing showed that it is impossible for any computer C to correctly answer the question of "will this program halt?" for every possible program. The reason is that we can always construct an adversarial program—itself a program that C should be able to predict—that intentionally does the opposite of what C expects and thus causes C itself to halt [WHP22].
Computer scientists thus say that the halting problem is undecidable over all Turing machines. Again, it's not that the halting problem is merely hard for computers, it simply cannot be answered in every conceivable case.
In fact we can see impossibility up and down the Chomsky hierarchy (Table 3). For instance, it is impossible for a finite state automaton (Type-3) to correctly reverse words of every possible length (e.g. convert "bad" into "dab", but for arbitrarily long words). In order to reverse a string of any length we need a pushdown automaton (Type-2). (Confusingly, Type-0 is the top of the Chomsky hierarchy and Type-3 is the bottom. Also confusingly, Chomsky is a libertarian socialist, whatever that means.)
Indeed as we go up the Chomsky hierarchy, we find that every higher class of automaton can solve all of the problems of the lower classes, but the reverse is not true. We see the economic calculation problem as a problem for which there is no algorithmic or socialist solution. The distributed computation of the market is therefore a higher and stronger process than central planning.
Now we must be careful here. At no point have I said that the theorems of computer science apply to humans. Rather, the imaginary constructs of computer science can provide strong intuition as to why Austrian economists view socialist and market economies as categorically different. Mises writes:
“Socialism is an alternative to capitalism as potassium cyanide is an alternative to water.”
Mises does not compare socialism to cyanide as an insult, but to make a wertfrei, albeit witty, declaration of what is possible and what is not possible under different economic systems. Mises's work shows that economic calculation is not decidable by the algorithmic process of socialism.
That is to say, no planned economy can convert the subjective ordinals of personal value into objective market prices, for it lacks the engine of the market.
A judgment of value does not measure, it arranges in a scale of degrees, it grades. It is expressive of an order of preference and sequence, but not expressive of measure and weight. Only the ordinal numbers can be applied to it, but not the cardinal numbers. It is vain to speak of any calculation of values. Calculation is possible only with cardinal numbers. The difference between the valuation of two states of affairs is entirely psychical and personal. It is not open to any projection into the external world. It can be sensed only by the individual. It cannot be communicated or imparted to any fellow man. It is an intensive magnitude. —Ludwig von Mises [LM49]
The irreducibility of the market process
The market process cannot be substituted by central planning any more than water can be safely substituted by cyanide. In this sense the market process is irreducible. We know from the theory of cellular automata that certain processes, though well-defined and even simple in their construction, are believed to be not only unpredictable, but irreducible. The impossibility of the halting problem arises from the fact that some programs cannot be short-cut—one has to simply run the programs and only then discover where they lead. Stephen Wolfram argues—but as yet there is no definitive proof—that certain highly simple machines, much like the Boids we met earlier, cannot be short-cut. There is no way to "jump ahead" and predict where the process will be after some time. One is forced to simply run the program and find out.
Consider proof of work in Bitcoin. Miners value SHA-256 hashes as proof of work not because gigawatts of electricity were expended to find them, but because there is no known shortcut, no polynomial-time algorithm that can invert SHA-256. Thus proof of work does not espouse the meaningless labor theory of value :) After all, we could use computers to continually multiply 100 by itself, and consume mountains of electricity, but that work is trivial to short-cut: after n iterations the value is always 10 to the power of 2n. The reducibility of multiplying 100 by itself renders the process worthless.
Analogously, the Austrian economists argue that the market is irreducible: there is no bureaucratic or algorithmic shortcut that can discover market prices.
Central planning and the ludic fallacy
A central planner is a person or committee with a model of the world and a plan to reshape that world. But the models of central planners, like any maps, are not the territory. The real world, to say nothing of wholly unpredictable human action, is too vast and dimensional to be compressed into any mind, committee, or machine. As we'll demonstrate with the COVID pandemic, central planners therefore address not complex realities, but oversimple caricatures of reality. Taleb calls this error the ludic fallacy, from the Greek word for "games". Central planners mistake toy models for the "ecologically complex real world" [NT09]. The ludic fallacy is reflected in a variety of aphorisms:
- The menu is not the meal
- The map is not the territory
- The corpse is not the creature (Goethe on his butterfly collection)
- All models are wrong, but some are useful (from statistician George Box)
All models are wrong, but some are useful
In the theory of computation the above are not just aphorisms but inescapable limits to any and every conceivable model. Models, we must note, are simply algorithms in the formal sense of the word. To take an example from the red hot field of machine learning (think "self-driving cars") we know that every model powerful enough to generalize intelligently is subject to inductive bias. Neural networks, for instance, are partial to hypotheses that can be found by gradient descent (the algorithm that they use to explore alternative hypotheses). Decision trees, yet another modeling algorithm, are partial to short trees. We can therefore see, and it has been proven mathematically, that every model that is powerful enough to be useful is biased. It is further the case that if we remove the bias of any model, we destroy its ability to generalize, a model without bias do nothing more than rote memorization. This is in spirit why Mises rejects historical facts as evidence for economic conclusions. For economists do not disagree on the facts of history, but disagree on the interpretation of those facts, an interpretation that pure praxeological reasoning alone can provide. The facts of history are broad and varied enough to be cherrypicked to support virtually any economic theory. The truth of praxeological reasoning is therefore a function of the internal consistency of its arguments, and requires no empirical justification.
Computer scientists sometimes refer to the fact that "all models are wrong" as the No Free Lunch (NFL) theorem. Just as inductive bias implies that every model has a blindspot, the NFL theorem states that every model that outperforms in one domain must underperform in another. Said another way, all algorithms are equally bad (or equally good) if you average their performance over the space of all possible problems. If you've ever played Dungeons and Dragons, or World of Warcraft, it's rarely possible to have a character that is perfect in every attribute. Rather, each character is specialized to be high in some attributes and low in other attributes. So, for instance, super strong characters might be dumb. Or super smart characters might be weak. A diverse division of labor. But, on average, every character is as good at as many tasks as they are bad at, and that is the No Free Lunch theorem in a nutshell.
The General Theory of physics envy
In the realm of economics, perhaps no ludic fallacy has been as harmful as the work of John Maynard Keynes. Keynes greatly admired Sir Isaac Newton, who contributed the vaunted laws of physical motion as equations. One hears physics envy in Keynes's concepts of leakages and injections, as if the economy were an inflatable balloon. Keynes's master work, The General Theory of Employment, Interest and Money gestures in the very title to steal the prestige of Einstein's General Theory of Relativity—but the similarities end at the titles of these books. Although Keynes authored the General Theory around three equations in three variables, the mere presence of mathematics doesn't make his conclusions true. Mises pulls no punches in declaring that Keynes sold inflationist snake oil in a fancy bottle with equations on the label:
Keynes did not add any new idea to the body of inflationist fallacies, a thousand times refuted by economists. His teachings were even more contradictory and inconsistent than those of his predecessors who, like Silvio Gesell, were dismissed as monetary cranks. He merely knew how to cloak the plea for inflation and credit expansion in the sophisticated terminology of mathematical economics. The interventionist writers were at a loss to advance plausible arguments in favor of the policy of reckless spending; they simply could not find a case against the economic theorem concerning institutional unemployment. [LM49]
Here we see the methodological error of Keynes in the eyes of the Austrian economists. First, Keynes sought to apply physical models with constant relations to unpredictable and purely variable human action:
The exchange ratios which we have to deal with are permanently fluctuating. There is nothing constant and invariable in them. They defy any attempt to measure them. They are not facts in the sense in which a physicist calls the establishment of the weight of a quantity of copper a fact. They are historical events, expressive of what happened once at a definite instant and under definite circumstances. —Ludwig von Mises [LM49]
Second, Keynes models human action from the top down. He proceeds from the complex economy down to its effect upon the only factor that matters, acting humans. Had Keynes instead started with the axiom of action and worked his way up to the principles of society, he might have realized that economic phenomena are uncertain enough to defy any equation. For the praxeologist it is folly to believe that a dynamic and organic economy, under the continuous and disruptive force of creative destruction by entrepreneurs, would ever stand still long enough for Keynes's theoretical formulas to have even a chance to be accurate.
But the crime of the ludic fallacy goes far beyond Keynes and the General Theory. A model is a dangerous thing to have, for it emboldens bureaucrats with the pretense of knowledge. The more empirical evidence the model seems to have, the bolder the risks central planners will take under its banner. The fall of Long-Term Capital Management, run by Nobel-prize-winning economists who mistook their Gaussian models to be accurate in a non-Gaussian world, shows that even the most learned actors will cleave to false models until economic death does them part.
A unified theory of socialism, capitalism, and when they work
Wertfrei, socialism and central planning aren't bad. Rather, there are fixed and humble limits as to when and how socialist or command economies, as distinguished from market economies, can succeed. We will illustrate not only the conditions under which socialist central planning fails, but the limited conditions under which it can succeed. For example, socialist policies are workable and even desirable at intimate scales, such as the nuclear family. Even the staunchest libertarian—one who calmly watches government agencies and communist nations fail without lifting a finger—is loathe to let his parents, children, or siblings go hungry and naked. Nevertheless, the same socialist policies that sustain at the family level, implode at the national level. What emerges is a diagonal along which information—the fuel of economic calculation—diverges and decentralizes.
We can conceive of central planning as a viable strategy for small, tight-knit units such as individuals and nuclear families, where there is sufficient information and trust for a single decision to passably benefit the entire unit. Although families are exempt from the economic calculation problem, ever so slightly larger economies are not [MR91]. At social scales larger than the nuclear family, human diversity quickly renders averages, aggregates, and consensus—the currency of central planners—into blunt instruments that obscure variegated realities under "one size fits none" policies.
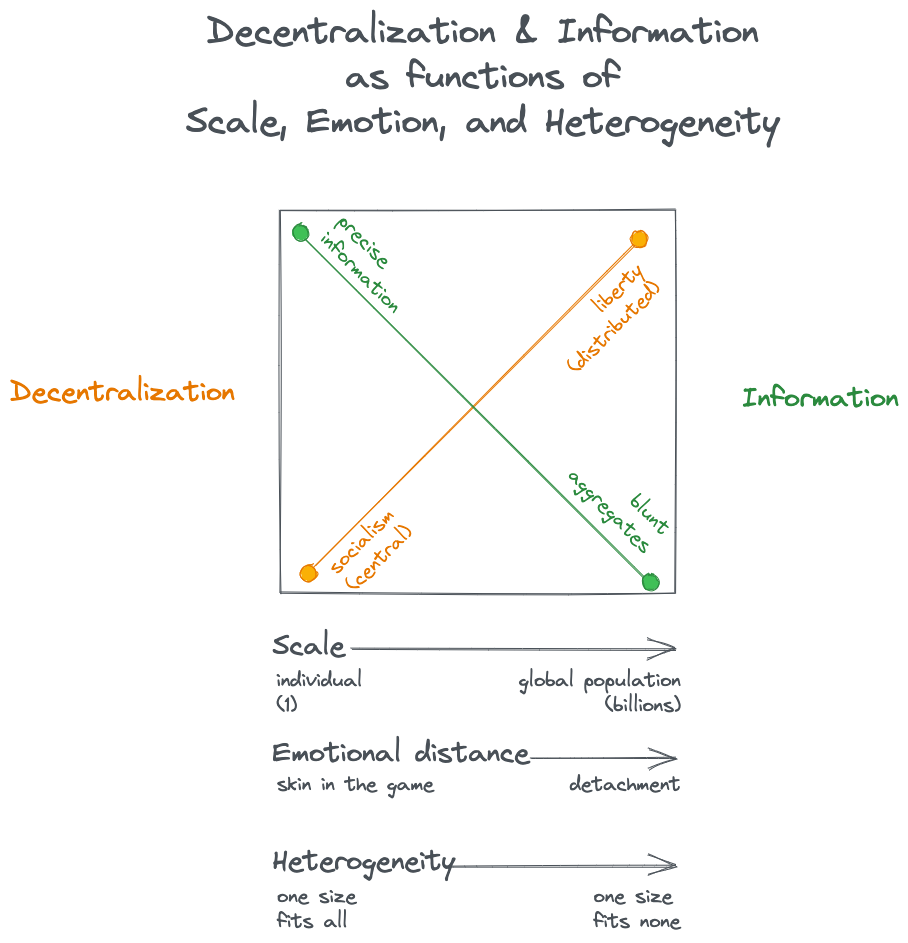
As shown in Figure 4, the need for decentralization varies inversely with the availability of precise and personalized information. As the scale, emotional distance, and demographic heterogeneity of human populations increase, central planning fails to compute a passably optimal distribution of scarce resources with alternate uses. Emotional distance is in some sense the opposite of skin in the game. Skin in the game entails conditions under which the bearer suffers direct loss for misjudging the effects of his actions (and often also reaps a direct reward for correctly judging the same). When emotional distance is low, such as with ourselves and our immediate family, skin in the game is correspondingly high.
Democrats need to learn experts aren’t always right
— Balaji Srinivasan (@balajis) May 12, 2020
Republicans need to learn experts aren’t always wrong
Libertarians need to learn that a state can succeed
Progressives need to learn that a state can fail
Humans are not fungible
To demonstrate the effects of scale, skin in the game, and heterogeneity on the feasibility of central planning and the actionability of information, I have taken the intellectual liberty of referring to individuals as "economies" that can be more or less socialist in nature. I present these one-person economies first as a thought experiment in what Mises calls autistic exchange (Robinson-Crusoe-like economies of a single person), and second to illustrate a fundamental error of socialism, the mistaken belief that human beings are fungible.
Fungible entities are sufficiently equivalent to be seamlessly interchanged. To a limited degree, standardized commodities like gold, oil, and wheat are fungible. But humans are never fungible. No matter how noble they may be in precept, no person can in practice regard all human beings as truly equal in value. Self-introspection reveals that, under conditions of comparable need, one cannot regard an anonymous homeless woman in a foreign country as equally worthy of charity as one's own mother. Moreover, in the course of evolution, fitness demands preferential treatment for blood relatives over strangers (just as biological theories of altruism in proportion to genetic similarity demonstrate).
Marx declared the ethos of socialism as "From each according to his ability, to each according to his needs." It should now be clear through praxeological arguments that Marx's maxim, no matter how noble it sounds, is impossible in practice. It is both natural and expedient—I did not say "right"—for you to regard the loss of your pinky finger with greater severity than casualties of war halfway around the globe. Adam Smith captures this truth in his Theory of Moral Sentiments:
If he was to lose his little finger to-morrow, he would not sleep to-night; but, provided he never saw them, he will snore with the most profound security over the ruin of a hundred millions of his brethren, and the destruction of that immense multitude seems plainly an object less interesting to him, than this paltry misfortune of his own. [ASTMS]
This is not to say that humans are unwilling or incapable of altruism or Christ-like sacrifice. Indeed they are. And Smith goes on to vindicate the same. The enduring point is that acting humans cannot regard all humans as equal and fungible. Ironically, the fact that altruism and self-sacrifice predominate at the level of the nuclear family predisposes us to believe in socialism. Based on our very own familial experiences of altruism, love, and generosity, it seems that socialism should be possible at the national level. But this is a category error that falsely conflates families with nations. I have therefore introduced scale, emotional distance, and heterogeneity as factors that directly impair the feasibility of central planning (Figure 4).
It is instructive to observe that small and culturally homogenous nations (South Korea, Taiwan), or small nations (New Zealand), or large but homogenous nations (China) have had relatively more success in addressing the pandemic with central planning—to say nothing of their despotic means. Whereas a large and heterogenous nation like the United States, with a culture of individualism to boot, can never expect to succeed with central planning.
COVID, an object lesson in central planning
The general failure of worldwide governments to overcome the COVID-19 pandemic is a failure of information. Central planning on behalf of millions of citizens necessarily relies on aggregations, averages, and models that compress diverse populations into flat numbers. By falsely regarding a large and demographically diverse populace as uniform "machinery"—Supreme Court Justice Sotomayor's metaphor for humans infected with a virus—lockdowns have inflicted significant harm in the form of poverty, suicide, domestic abuse, depression, and untreated ailments. The early epidemiological models of masks, transmission, immunity, and fatality were so wrong as to require retractions and 180° turns from the CDC, WHO, and the US's nationally-appointed experts.
Stanford's Jay Bhattacharya—a physician, economist, and epidemiologist—contends, along with his eminent coauthors and numerous co-signatories of the The Great Barrington Declaration, that the only reasonable response to the pandemic is focused protection that takes into account different risk factors for different individuals. The variable of age, followed distantly by the variable of obesity, reveals vastly different risks for different demographics. Per the CDC's best estimates, the infection fatality rate for COVID-19 ranges from .002% (20 in 1-million) for persons under 18, to 9% for persons over 64 (90,000 in 1-million)—a staggering 4,500-fold difference in fatality risk. Blindly locking down entire populations based on such a dynamic range of risk profiles is "one size fits none" personified.
Before we abandon the controversy of COVID policies, the rebel in me must remark on the central planners' concept of "essential jobs." At the onset of the pandemic, certain workers were labeled "essential" and permitted to continue work, while others were ordered to stay home. In spite of this trite label, logic dictates that for every individual whose job puts food on the table, his or her job is literally essential. Only an emotionally and informationally distant bureaucrat could forget this fact and mandate the opposite, harming first and foremost the marginalized demographics that hover just above poverty.
Authoritarianism is a contagious disease
Naysayers will respond that the contagiousness of COVID-19 makes it categorically different from non-contagious risks like cancer and car accidents. A sweeping and uncompromising policy response, they argue, is therefore necessary. To this objection we may reply that authoritarianism is highly contagious and deleterious to human health, as evidenced by mass graves in the USSR, China, Cambodia, Germany, and North Korea. Even if we callously dismiss the body counts of socialist regimes as "not real socialism", controlled statistical evidence shows that highly similar demographic groups are wealthier and healthier under market economies than they are under socialism. (See [DU19] for data on Scandinavians living in the US versus in Scandinavia.)
The ratchet of bureaucracy
The contagiousness of authoritarianism is a ratchet effect, a kind of bureaucratic inertia: a government in motion tends to stay in motion (with permission, denial, and policing). The threat of authoritarianism merits as much precaution and prevention as any pandemic. Not unlike viruses, government initiatives tend to expand their reach over time. In stark contrast to private agencies that go bankrupt and vanish into history, government initiatives that flounder are precisely the initiatives that attract higher funding and expand. Ruing this perverse dynamic, Milton Friedman wrote that
Nothing is so permanent as a temporary government program.
America's incessant trillion-dollar wars on nouns like Poverty, Drugs, Terror, and COVID demonstrate not only continued growth in spite of objective failure, but unforeseen and ironic consequences to intervention. History is witness to hundreds of such cobra effects, named for a law imposed in India during British rule that offered a bounty for dead cobras. The effect of India's cobra law was the opposite of its intent: Delhi's cobra population exploded as subjects bred cobras to mine bounties from the British Raj. In a sweeping and empirical analysis of how the government policies in the U.S. have not only failed but actively hurt the people they intended to save, Davies and Harrigan write in Cooperation and Coercion:
The problem is that imagining outcomes ignores the fact that people don’t respond to laws. People respond to incentives... Part of the reason that almost thirty million Americans remain uninsured even after the Affordable Care Act established a fine for being uninsured is that the cost of the fine is less than the cost of purchasing insurance. Finally, as numerous examples of unintended consequences demonstrate, even if we coerce people into altering their behaviors, we may end up causing them to do other things we like even less. —Davies & Harrigan [CC20]
Central planning destroys information
Our COVID digression had nothing to do with with COVID per se, and everything to do with the limits of central planning. As Hayek observed, the information that individuals possess supersedes the quantity and quality of information that any central planner could possibly obtain.
The market is the only known method of providing information enabling individuals to judge comparative advantages of different uses of resources of which they have immediate knowledge and through whose use, whether they so intend or not, they serve the needs of distant unknown individuals. This dispersed knowledge is essentially dispersed, and cannot possibly be gathered together and conveyed to an authority charged with the task of deliberately creating order. —Friedrich Hayek [FH11]
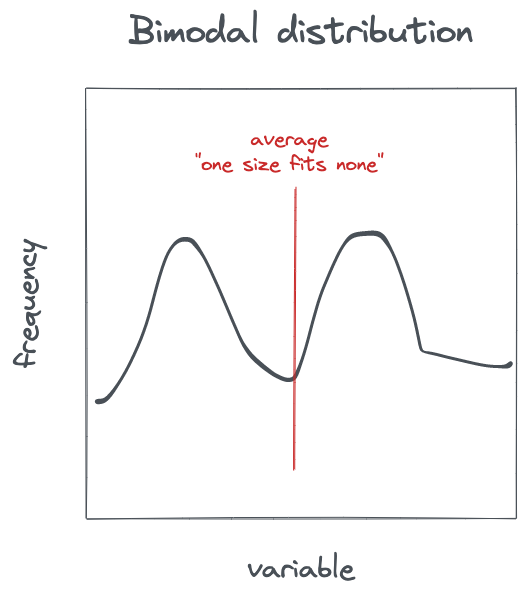
To appreciate the limits of information that reaches the desks of central planners, consider a demographically diverse populace that exhibits a bimodal distribution—a common occurrence in the real world (Figure 2). The variable in question could be age, obesity, income—it makes no difference. What matters is that the numbers that inform central planning collapse multi-dimensional realities down to one-dimensional scalars. In the case of our fictional bimodal distribution, the average is not just a poor representation of the population, it is optimally bad in that it literally represents the least number of people. Far from its democratic and egalitarian intentions, central planning actually produces "one size fits none" results for large, diverse, and emotionally distant populations.
Shared ownership in name only
Proponents of socialism might object that central planners are thinking, feeling human beings with a sense of duty towards their fellow man. But recall that any central plan that is not algorithmic cannot be fair, for it relies on the whims of the socialist planners themselves. Hoppe dissects the subtlety of nominal versus practical ownership of the means of production, distribution, and exchange as follows:
Declaring everybody a co-owner of everything [socialism] solves the problem of differences in ownership only nominally. It does not solve the real underlying problem: differences in the power to control. —Hans-Hermann Hoppe [HHTSC]
In spite of the ideological camouflage that socialism serves the "common good", in actual practice only a small number of privileged party insiders exercise power. Fidel Castro owned dozens of mansions and suffered none of the privations of hunger, poverty, and torture that plague common Cubans [JS14]. In a market economy the consumers are kings, for their needs guide production through the process of the market. Socialism substitutes rule by the demands of the consumers with rule by a cabal who, no matter how noble, must ultimately make singular tradeoffs that prefer their limited knowledge over the dispersed knowledge of the populace, and that trade the benefits of their cabal against the benefits of the governed. Even the devout socialist knows that real relations trump imaginary ones. Mises thus chides that
The worst thing that can happen to a socialist is to have his country ruled by socialists who are not his friends.
Acknowledgements
Robert Breedlove engaged in early conversations on central planning and Austrian economics that would become this article, and provided detailed feedback on early drafts. Balaji Srinivasan provided constructive feedback on cases where central planning works, leading to a more subtle understanding of heterogeneity. Dr. Richard Stark first introduced me to the wonders of the theory of computation and the Chomsky hierarchy.
References
- NT12 Antifragile: things that gain from disorder, Nassim Taleb, 2012.
- MF79 Free to Choose: A Personal Statement, Milton Friedman, 1979.
- LM49 Human Action: A Treatise on Economics, Ludwig von Mises, 1949.
- FH01 The Road to Serfdom, the Definitive Edition: Text and Documents, Friedrich A. Hayek, 2001 (original from 1944).
- FH11, The Fatal Conceit: The Errors of Socialism, Friedrich A. Hayek, 2011.
- FH45, The use of knowledge in Society, Friedrich A. Hayek, 1945.
- DU15 — Debunking Utopia: Exposing the Myth of Nordic Socialism, Nima Sanandaji, 2016.
- Wi14 The Questions That Computers Can Never Answer, Wired, 2014.
- MR91, The End of Socialism and the Calculation Debate Revisited, The Review of Austrian Economics 5, no. 2 1991, Murray N. Rothbard
- JS14, The Double Life of Fidel Castro: My 17 Years as Personal Bodyguard to El Lider Maximo, Juan Reinaldo Sanchez, Axel Gyldén, et. al
- CC20, Cooperation and Coercion: How Busybodies Became Busybullies and What that Means for Economics and Politics , Antony Davies & James R. Harrigan
- HHTSC, A Theory of Socialism and Capitalism, Hans-Hermann Hoppe, 1988.
- LM22, The Impossibility of Economic Calculation under Socialism, Ludwig von Mises, Online Library of Liberty.
- GS13, Ludwig von Mises and Economic Calculation Under Socialism, Libertarianism.org
- AT19, The Murmurations of Starlings, Alan Taylor, The Atlantic, 2019.
- MR19, Praxeology: The Methodology of Austrian Economics, mises.org, Murray N. Rothbard
- BA19, Complexity Economics: Proceedings of the Santa Fe Institute's 2019 Fall Symposium, W. Brian Arthur (Author), Eric D. Beinhocker (Editor), Allison Stanger (Editor), 2019.
- JB99, On the Complexities of Complex Economic Dynamics, Journal of Economic Perspectives—Volume 13, Number 4—Fall 1999—Pages 169–192, J. Barkley Rosser Jr.
- CE11, The Complexity Era in Economics, Review of Political Economy, 23:3, 357-369, DOI: 10.1080/09538259.2011.583820, Richard P. F. Holt , J. Barkley Rosser Jr. & David Colander, 2011.
- [LRI15], I, Pencil, Leondard Read, Foundation for Economic Education (fee.org), 2015.
- WEPDA22, Effect of psychoactive drugs on animals, Wikipedia, 2022.
- WFS22, Formal aystem, Wikipedia, 2022.
- WPV22, Paradox of value, Wikipedia, 2022.
- WB22, Boids, Wikipedia, 2022.
- WCA22, Cellular automata, Wikipedia 2022.
- WHP22, Halting problem, Wikipeda, 2022.
- WCH, Chomsky hierarchy, Wikipedia, 2022.
- WCS22, Complex system, Wikipedia, 2022.
- WECP22, Economic calculation problem, Wikipedia, 2022.
